Find the General Solution of 2y 7y 4y 4y 0

17.E: Second-Order Differential Equations (Exercises)

1. Last updated

2. Save as PDF

Page ID

3607

• 
• Professor (Mathematics) at Massachusetts Institute of Technology (Strang) & University of Wisconsin-Stevens Point (Herman)
• Publisher: OpenStax CNX

17.1: Second-Order Linear Equations

Q17.1.1

Classify each of the following equations as linear or nonlinear. If the equation is linear, determine whether it is homogeneous or nonhomogeneous.

1. \(x^3y''+(x-1)y'-8y=0\)
2. \((1+y^2)y''+xy'-3y= \cos x\)
3. \(xy''+e^yy'=x\)
4. \(y''+ \frac{4}{x}y'-8xy=5x^2+1 \)
5. \(y''+( \sin x)y'-xy=4y \)
6. \(y''+(\frac{x+3}{y})y'=0\)

Q17.1.2

For each of the following problems, verify that the given function is a solution to the differential equation. Use a graphing utility to graph the particular solutions for several values of c ₁ and c ₂. What do the solutions have in common?

1. [T] \(y''+2y'-3y=0; ~~ y(x)=c_1e^x+c_2e^{-3x}\)
2. [T]\(x^2y''-2y-3x^2+1=0; ~~y(x)=c_1x^2+c_2x^{-1}+x^2 \ln(x)+ \frac{1}{2} \)
3. [T] \(y''+14y+49y=0;y″+14y′+49y=0; y(x)=c1e−7x+c2xe−7xy(x)=c1e−7x+c2xe−7x
4. [T]6y''−49y′+8y=0;6y″−49y′+8y=0; y(x)=c1ex/6+c2e8xy(x)=c1ex/6+c2e8x

Q17.1.3

Find the general solution to the linear differential equation.

y''−3y′−10y=0y″−3y′−10y=0

y''−7y′+12y=0y″−7y′+12y=0

y''+4y′+4y=0y″+4y′+4y=0

4y''−12y′+9y=04y″−12y′+9y=0

2y''−3y′−5y=02y″−3y′−5y=0

3y''−14y′+8y=03y″−14y′+8y=0

y''+y′+y=0y″+y′+y=0

5y''+2y′+4y=05y″+2y′+4y=0

y''−121y=0y″−121y=0

8y''+14y′−15y=08y″+14y′−15y=0

y''+81y=0y″+81y=0

y''−y′+11y=0y″−y′+11y=0

2y''=02y″=0

y''−6y′+9y=0y″−6y′+9y=0

3y''−2y′−7y=03y″−2y′−7y=0

4y''−10y′=04y″−10y′=0

36d2ydx2+12dydx+y=036d2ydx2+12dydx+y=0

25d2ydx2−80dydx+64y=025d2ydx2−80dydx+64y=0

d2ydx2−9dydx=0d2ydx2−9dydx=0

4d2ydx2+8y=04d2ydx2+8y=0

Q17.1.4

Solve the initial-value problem.

y''+5y′+6y=0,y(0)=0,y′(0)=−2y″+5y′+6y=0,y(0)=0,y′(0)=−2

y''+2y′−8y=0,y(0)=5,y′(0)=4y″+2y′−8y=0,y(0)=5,y′(0)=4

y''+4y=0,y(0)=3,y′(0)=10y″+4y=0,y(0)=3,y′(0)=10

y''−18y′+81y=0,y(0)=1,y′(0)=5y″−18y′+81y=0,y(0)=1,y′(0)=5

y''−y′−30y=0,y(0)=1,y′(0)=−16y″−y′−30y=0,y(0)=1,y′(0)=−16

4y''+4y′−8y=0,y(0)=2,y′(0)=14y″+4y′−8y=0,y(0)=2,y′(0)=1

25y''+10y′+y=0,y(0)=2,y′(0)=125y″+10y′+y=0,y(0)=2,y′(0)=1

y''+y=0,y(π)=1,y′(π)=−5y″+y=0,y(π)=1,y′(π)=−5

Solve the boundary-value problem, if possible.

y''+y′−42y=0,y(0)=0,y(1)=2y″+y′−42y=0,y(0)=0,y(1)=2

9y''+y=0,y(3π2)=6,y(0)=−89y″+y=0,y(3π2)=6,y(0)=−8

y''+10y′+34y=0,y(0)=6,y(π)=2y″+10y′+34y=0,y(0)=6,y(π)=2

y''+7y′−60y=0,y(0)=4,y(2)=0y″+7y′−60y=0,y(0)=4,y(2)=0

y''−4y′+4y=0,y(0)=2,y(1)=−1y″−4y′+4y=0,y(0)=2,y(1)=−1

y''−5y′=0,y(0)=3,y(−1)=2y″−5y′=0,y(0)=3,y(−1)=2

y''+9y=0,y(0)=4,y(π3)=−4y″+9y=0,y(0)=4,y(π3)=−4

4y''+25y=0,y(0)=2,y(2π)=−24y″+25y=0,y(0)=2,y(2π)=−2

Find a differential equation with a general solution that is y=c1ex/5+c2e−4x.y=c1ex/5+c2e−4x.

Q17.1.X

Find a differential equation with a general solution that is y=c1ex+c2e−4x/3.y=c1ex+c2e−4x/3.

For each of the following differential equations:

1. Solve the initial value problem.
2. [T] Use a graphing utility to graph the particular solution.

y''+64y=0;y(0)=3,y′(0)=16y″+64y=0;y(0)=3,y′(0)=16

y''−2y′+10y=0y(0)=1,y′(0)=13y″−2y′+10y=0y(0)=1,y′(0)=13

y''+5y′+15y=0y(0)=−2,y′(0)=7y″+5y′+15y=0y(0)=−2,y′(0)=7

Q17.1.X

(Principle of superposition) Prove that if y1(x)y1(x) and y2(x)y2(x) are solutions to a linear homogeneous differential equation, y''+p(x)y′+q(x)y=0,y″+p(x)y′+q(x)y=0, then the function y(x)=c1y1(x)+c2y2(x),y(x)=c1y1(x)+c2y2(x), where c1c1 and c2c2 are constants, is also a solution.

Prove that if a, b, and c are positive constants, then all solutions to the second-order linear differential equation ay''+by′+cy=0ay″+by′+cy=0 approach zero as x→∞.x→∞. (Hint: Consider three cases: two distinct roots, repeated real roots, and complex conjugate roots.)

17.2: Nonhomogeneous Linear Equations

Solve the following equations using the method of undetermined coefficients.

\(2y''−5y′−12y=6\)

\(3y''+y′−4y=8\)

\(y=c_1e^{−4x/3}+c_2e^x−2\)

\(y''−6y′+5y=e^{−x}\)

\(y''+16y=e^{−2x}\)

\(y=c_1 \cos4x+c_2 \sin 4x+\frac{1}{20}e^{−2x}\)

\(y″−4y=x^2+1\)

\(y″−4y′+4y=8x^2+4x\)

\(y=c_1e^{2x}+c_2xe^{2x}+2x^2+5x\)

\(y″−2y′−3y= \sin 2x\)

\(y″+2y′+y= \sin x+ \cos x\)

\(y=c_1e^{−x}+c_2xe^{−x}+\frac{1}{2} \sin x−\frac{1}{2} \cos x\)

\(y″+9y=e^x \cos x\)

\(y″+y=3 \sin 2x+x \cos 2x\)

\(y=c_1 \cos x+ c_2 \sin x−\frac{1}{3}x \cos 2x−\frac{5}{9} \sin 2x\)

\(y″+3y′−28y=10e{4x}\)

\(y″+10y′+25y=xe^{−5x}+4\)

\(y=c_1e^{−5x}+c_2xe^{−5x}+\frac{1}{6}x^3e^{−5x}+\frac{4}{25}\)

In each of the following problems,

1. Write the form for the particular solution \(y_p(x)\) for the method of undetermined coefficients.
2. [T] Use a computer algebra system to find a particular solution to the given equation.

\(y″−y′−y=x+e^{−x}\)

\(y″−3y=x^2−4x+11\)

a. \(y_p(x)=Ax^2+Bx+C\)

b. \(y_p(x)=−\frac{1}{3}x^2+\frac{4}{3}x−\frac{35}{9}\)

\(y''−y′−4y=e^x \cos 3x \)

\(2y″−y′+y=(x^2−5x)e^{−x}\)

a. \(y_p(x)=(Ax^2+Bx+C)e^{−x}\)

b. \(y_p(x)=(\frac{1}{4}x^2−\frac{5}{8}x−\frac{33}{32})e^{−x}\)

\(4y″+5y′−2y=e^{2x}+x \sin x\)

\(y''−y′−2y=x^2e^x \sin x\)

a. \(y_p(x)=(Ax^2+Bx+C)e^x \cos x+(Dx^2+Ex+F)e^x \sin x\)

b. \(y_p(x)=(−\frac{1}{10}x^2−\frac{11}{25}x−\frac{27}{250})e^x \cos x +(−\frac{3}{10}x^2+\frac{2}{25}x+\frac{39}{250})e^x \sin x\)

Solve the differential equation using either the method of undetermined coefficients or the variation of parameters.

\(y″+3y′−4y=2e^x\)

\(y''+2y′=e^{3x}\)

\(y=c_1+c_2e^{−2x}+\frac{1}{15}e^{3x}\)

\(y''+6y′+9y=e^{−x}\)

\(y''+2y′−8y=6e^{2x}\)

\(y=c_1e^{2x}+c_2e^{−4x}+xe^{2x}\)

Solve the differential equation using the method of variation of parameters.

\(4y″+y=2 \sin x\)

\(y″−9y=8x\)

\(y=c_1e^{3x}+c_2e^{−3x}−\frac{8x}{9}\)

\(y″+y= \sec x, \;\;\;\;\;\;\;\;\;\;0<x<π/2\)

\(y″+4y=3 \csc 2x,\;\;\;\;\;\;\;\;\;\; 0<x<π/2\)

\(y=c_1 \cos 2x+c_2 \sin 2x−\frac{3}{2} x \cos 2x+\frac{3}{4} \sin 2x \ln ( \sin 2x)\)

Find the unique solution satisfying the differential equation and the initial conditions given, where \(y_p(x)\) is the particular solution.

\(y″−2y′+y=12e^x,y_p(x)=6x^2e^x, y(0)=6,y′(0)=0\)

\(y''−7y′=4xe^{7x},y_p(x)=\frac{2}{7}x^2e^{7x}−\frac{4}{49}xe^{7x},y(0)=−1,y'(0)=0\)

\(y=− \frac {347}{343}+ \frac {4}{343}e^{7x}+\frac{2}{7}x^2e^{7x}−\frac{4}{49}xe^{7x}\)

\(y″+y= \cos x−4 \sin x, y_p(x)=2x \cos x+\frac{1}{2} x \sin x, y(0)=8,y′(0)=−4\)

\(y″−5y′=e^{5x}+8e^{−5x}, y_p(x)=\frac{1}{5}xe^{5x}+\frac{4}{25}e^{−5x}, y(0)=−2,y′(0)=0\)

\(y=−\frac{57}{25}+\frac{3}{25}e^{5x}+\frac{1}{5}xe^{5x}+\frac{4}{25}e^{−5x}\)

In each of the following problems, two linearly independent solutions—\(y_1\) and \(y_2\)—are given that satisfy the corresponding homogeneous equation. Use the method of variation of parameters to find a particular solution to the given nonhomogeneous equation. Assume x > 0 in each exercise.

\(x^2y″+2xy′−2y=3x, y_1(x)=x,y2(x)=x^{−2}\)

\(x^2y''−2y=10x^2−1,y_1(x)=x^2,y_2(x)=x^{−1}\)

\(y_p=\frac{1}{2}+\frac{10}{3}x^2 \ln x\)

17.3: Applications

A mass weighing 4 lb stretches a spring 8 in. Find the equation of motion if the spring is released from the equilibrium position with a downward velocity of 12 ft/sec. What is the period and frequency of the motion?

A mass weighing 2 lb stretches a spring 2 ft. Find the equation of motion if the spring is released from 2 in. below the equilibrium position with an upward velocity of 8 ft/sec. What is the period and frequency of the motion?

\(x″+16x=0, x(t)=\frac{1}{6} \cos (4t)−2 \sin (4t),\) period \(=\frac{π}{2} \text{sec},\) frequency \(=\frac{2}{π} \text{Hz}\)

A 100-g mass stretches a spring 0.1 m. Find the equation of motion of the mass if it is released from rest from a position 20 cm below the equilibrium position. What is the frequency of this motion?

A 400-g mass stretches a spring 5 cm. Find the equation of motion of the mass if it is released from rest from a position 15 cm below the equilibrium position. What is the frequency of this motion?

\(x″+196x=0, x(t)=0.15 \cos (14t),\) period \(=\frac{π}{7} \text{sec},\) frequency \(=\frac{7}{π} \text{Hz}\)

A block has a mass of 9 kg and is attached to a vertical spring with a spring constant of 0.25 N/m. The block is stretched 0.75 m below its equilibrium position and released.

1. Find the position function \(x(t)\) of the block.
2. Find the period and frequency of the vibration.
3. Sketch a graph of \(x(t)\).
4. At what time does the block first pass through the equilibrium position?

A block has a mass of 5 kg and is attached to a vertical spring with a spring constant of 20 N/m. The block is released from the equilibrium position with a downward velocity of 10 m/sec.

1. Find the position function \(x(t)\) of the block.
2. Find the period and frequency of the vibration.
3. Sketch a graph of \(x(t)\).
4. At what time does the block first pass through the equilibrium position?

a. \(x(t)=5 \sin (2t)\)

b. period \(=π \text{sec},\) frequency \(=\frac{1}{π} \text{Hz}\) c. 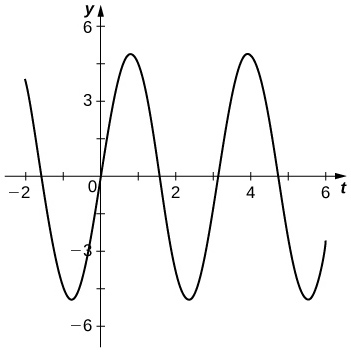d. \(t=\frac{π}{2} \text{sec}\)

A 1-kg mass is attached to a vertical spring with a spring constant of 21 N/m. The resistance in the spring-mass system is equal to 10 times the instantaneous velocity of the mass.

1. Find the equation of motion if the mass is released from a position 2 m below its equilibrium position with a downward velocity of 2 m/sec.
2. Graph the solution and determine whether the motion is overdamped, critically damped, or underdamped.

An 800-lb weight (25 slugs) is attached to a vertical spring with a spring constant of 226 lb/ft. The system is immersed in a medium that imparts a damping force equal to 10 times the instantaneous velocity of the mass.

1. Find the equation of motion if it is released from a position 20 ft below its equilibrium position with a downward velocity of 41 ft/sec.
2. Graph the solution and determine whether the motion is overdamped, critically damped, or underdamped.

a. \(x(t)=e^{−t/5}(20 \cos (3t)+15 \sin(3t))\)

b. underdamped

A 9-kg mass is attached to a vertical spring with a spring constant of 16 N/m. The system is immersed in a medium that imparts a damping force equal to 24 times the instantaneous velocity of the mass.

1. Find the equation of motion if it is released from its equilibrium position with an upward velocity of 4 m/sec.
2. Graph the solution and determine whether the motion is overdamped, critically damped, or underdamped.

A 1-kg mass stretches a spring 6.25 cm. The resistance in the spring-mass system is equal to eight times the instantaneous velocity of the mass.

1. Find the equation of motion if the mass is released from a position 5 m below its equilibrium position with an upward velocity of 10 m/sec.
2. Determine whether the motion is overdamped, critically damped, or underdamped.

a. \(x(t)=5e^{−4t}+10te^{−4t}\)

b. critically damped

A 32-lb weight (1 slug) stretches a vertical spring 4 in. The resistance in the spring-mass system is equal to four times the instantaneous velocity of the mass.

1. Find the equation of motion if it is released from its equilibrium position with a downward velocity of 12 ft/sec.
2. Determine whether the motion is overdamped, critically damped, or underdamped.

A 64-lb weight is attached to a vertical spring with a spring constant of 4.625 lb/ft. The resistance in the spring-mass system is equal to the instantaneous velocity. The weight is set in motion from a position 1 ft below its equilibrium position with an upward velocity of 2 ft/sec. Is the mass above or below the equation position at the end of \(π\) sec? By what distance?

\(x(π)=\frac{7e^{−π/4}}{6}\) ft below

A mass that weighs 8 lb stretches a spring 6 inches. The system is acted on by an external force of \(8 \sin 8t \)lb. If the mass is pulled down 3 inches and then released, determine the position of the mass at any time.

A mass that weighs 6 lb stretches a spring 3 in. The system is acted on by an external force of \(8 \sin (4t) \) lb. If the mass is pulled down 1 inch and then released, determine the position of the mass at any time.

\(x(t)=\frac{32}{9} \sin (4t)+ \cos (\sqrt{128}t)−\frac{16}{9\sqrt{2}} \sin (\sqrt{128}t)\)

Find the charge on the capacitor in an RLC series circuit where \(L=40\) H, \(R=30Ω\), \(C=1/200\) F, and \(E(t)=200\) V. Assume the initial charge on the capacitor is 7 C and the initial current is 0 A.

Find the charge on the capacitor in an RLC series circuit where \(L=2\) H, \(R=24Ω,\) \(C=0.005\) F, and \(E(t)=12 \sin 10t\) V. Assume the initial charge on the capacitor is 0.001 C and the initial current is 0 A.

\(q(t)=e^{−6t}(0.051 \cos (8t)+0.03825 \sin (8t))−\frac{1}{20} \cos (10t)\)

A series circuit consists of a device where\(L=1\) H, \(R=20Ω,\) \(C=0.002\) F, and \(E(t)=12\) V. If the initial charge and current are both zero, find the charge and current at time t.

A series circuit consists of a device where \(L=12\) H, \(R=10Ω\), \(C=\frac{1}{50}\) F, and \(E(t)=250\) V. If the initial charge on the capacitor is 0 C and the initial current is 18 A, find the charge and current at time t.

\(q(t)=e^{−10t}(−32t−5)+5,I(t)=2e^{−10t}(160t+9)\)

17.4: Series Solutions of Differential Equations

Find a power series solution for the following differential equations.

\(y″+6y′=0\)

\(5y″+y′=0\)

\(y=c_0+5c_1 \sum_{n=1}^∞ \frac{(−x/5)^n}{n!}=c_0+5c_1e^{−x/5}\)

\(y''+25y=0\)

\(y''−y=0\)

\(y=c_0 \sum_{n=0}^∞ \frac{(x)^{2n}}{(2n)!}+c_1 \sum_{n=0}^∞ \frac{(x)^{2n+1}}{(2n+1)!}\)

\(2y′+y=0}

\(y′−2xy=0\)

\(y=c_0 \sum_{n=0}^∞ \frac{x^{2n}}{n!}=c_0e^{x2}\)

\((x−7)y′+2y=0\)

\(y''−xy′−y=0\)

\(y=c_0 \sum_{n=0}^∞ \frac{x^{2n}}{2^nn!}+c_1 \sum_{n=0}^∞ \frac{x^{2n+1}}{1⋅3⋅5⋅7⋯(2n+1)}\)

\((1+x^2)y''−4xy′+6y=0\)

\(x^2y''−xy′−3y=0\)

\(y=c_1x^3+\frac{c_2}{x}\)

\(y″−8y′=0, \; \; \; \; \; \; y(0)=−2,y′(0)=10\)

\(y″−2xy=0, \; \; \; \; \; \; y(0)=1,y′(0)=−3\)

\(y=1−3x+\frac{2x^3}{3!}−\frac{12x^4}{4!}+\frac{16x^6}{6!}−\frac{120x^7}{7!}+⋯\)

The differential equation \(x^2y″+xy′+(x^2−1)y=0\) is a Bessel equation of order 1. Use a power series of the form \(y=\sum_{n=0}^∞ a_nx^n\) to find the solution.

Chapter Review Exercises

True or False? Justify your answer with a proof or a counterexample.

If \(y\) and \(z\) are both solutions to \(y''+2y′+y=0,\) then \(y+z\) is also a solution.

True

The following system of algebraic equations has a unique solution:

\(\begin{align} 6z1+3z2 =8 \\ 4z1+2z2 =4. \end{align}\)

\(y=e^x \cos (3x)+e^x \sin (2x)\) is a solution to the second-order differential equation \(y″+2y′+10=0.\)

False

To find the particular solution to a second-order differential equation, you need one initial condition.

Classify the differential equation. Determine the order, whether it is linear and, if linear, whether the differential equation is homogeneous or nonhomogeneous. If the equation is second-order homogeneous and linear, find the characteristic equation.

\(y″−2y=0\)

second order, linear, homogeneous, \(λ^2−2=0\)

\(y''−3y+2y= \cos (t)\)

\((\frac{dy}{dt})^2+yy′=1\)

first order, nonlinear, nonhomogeneous

\(\frac{d^2y}{dt^2}+t \frac{dy}{dt}+sin^2 (t)y=e^t\)

For the following problems, find the general solution.

\(y''+9y=0\)

\(y=c_1 \sin (3x)+c_2 \cos (3x)\)

\(y''+2y′+y=0\)

\(y''−2y′+10y=4x\)

\(y=c_1e^x \sin (3x)+c_2e^x \cos (3x)+\frac{2}{5}x+\frac{2}{25}\)

\(y''= \cos (x)+2y′+y\)

\(y''+5y+y=x+e^{2x}\)

\(y=c_1e^{−x}+c_2e^{−4x}+\frac{x}{4}+\frac{e^{2x}}{18}−\frac{5}{16}\)

\(y''=3y′+xe^{−x}\)

\(y''−x^2=−3y′−\frac{9}{4}y+3x\)

\(y=c_1e^{(−3/2)x}+c_2xe^{(−3/2)x}+\frac{4}{9}x^2+\frac{4}{27}x−\frac{16}{27}\)

\(y''=2 \cos x+y′−y\)

For the following problems, find the solution to the initial-value problem, if possible.

\(y''+4y′+6y=0, y(0)=0, y′(0)=\sqrt{2}\)

\(y=e^{−2x} \sin (\sqrt{2}x)\)

\(y''=3y− \cos (x), y(0)=\frac{9}{4}, y′(0)=0\)

For the following problems, find the solution to the boundary-value problem.

\(4y′=−6y+2y″, y(0)=0, y(1)=1\)

\(y=\frac{e^{1−x}}{e^4−1}(e^{4x}−1)\)

\(y''=3x−y−y′, y(0)=−3, y(1)=0\)

For the following problem, set up and solve the differential equation.

The motion of a swinging pendulum for small angles \(θ\) can be approximated by \(\frac{d^2θ}{dt^2}+\frac{g}{L}θ=0,\) where \(θ\) is the angle the pendulum makes with respect to a vertical line, g is the acceleration resulting from gravity, and L is the length of the pendulum. Find the equation describing the angle of the pendulum at time \(t,\) assuming an initial displacement of \(θ_0\) and an initial velocity of zero.

\(θ(t)=θ_0 \cos (\sqrt{\frac{g}{l}}t)\)

The following problems consider the "beats" that occur when the forcing term of a differential equation causes "slow" and "fast" amplitudes. Consider the general differential equation \(ay″+by= \cos (ωt)\) that governs undamped motion. Assume that \(\sqrt{\frac{b}{a}}≠ω.\)

Find the general solution to this equation (Hint: call \(ω_0=\sqrt{b/a}\)).

Assuming the system starts from rest, show that the particular solution can be written as\(y=\frac{2}{a(ω_0^2−ω^2)} \sin (\frac{ω_0−ωt}{2}) \sin(\frac{ω_0+ωt}{2}).\)

[T] Using your solutions derived earlier, plot the solution to the system \(2y″+9y= \cos (2t)\) over the interval \(t=[−50,50].\) Find, analytically, the period of the fast and slow amplitudes.

For the following problem, set up and solve the differential equations.

An opera singer is attempting to shatter a glass by singing a particular note. The vibrations of the glass can be modeled by \(y″+ay= \cos (bt)\), where \(y''+ay=0\) represents the natural frequency of the glass and the singer is forcing the vibrations at \( \cos (bt)\).For what value bb would the singer be able to break that glass? (Note: in order for the glass to break, the oscillations would need to get higher and higher.)

\(b=\sqrt{a}\)

Find the General Solution of 2y 7y 4y 4y 0

Source: https://math.libretexts.org/Bookshelves/Calculus/Exercises_%28Calculus%29/Exercises:_Calculus_%28OpenStax%29/17.E:_Second-Order_Differential_Equations_%28Exercises%29

Share this post